PRAXIS: Extrema

Let \(f\) be the function defined by the graph below.

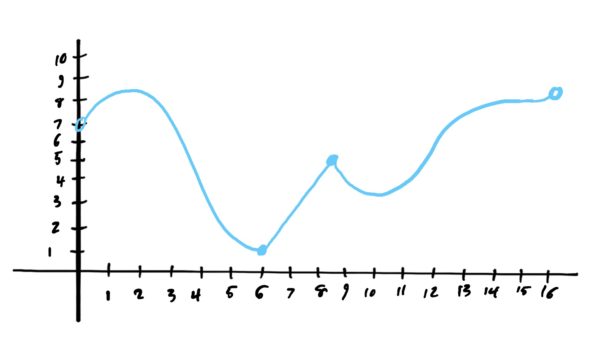
1. Find all local mins and maxes of \(f\).
2. What values does \(f\) attain at each of these local extrema?
3. What are the global maxes (if any)? What are the global mins (if any)?

Let \(g\) be defined by the graph below.

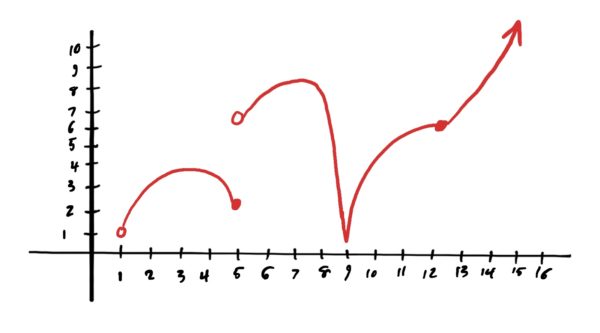
4. Find all local mins and maxes of \(f\).
5. What values does \(f\) attain at each of these local extrema?
6. What are the global maxes (if any)? What are the global mins (if any)?

Let \(h\) be defined by the graph below.

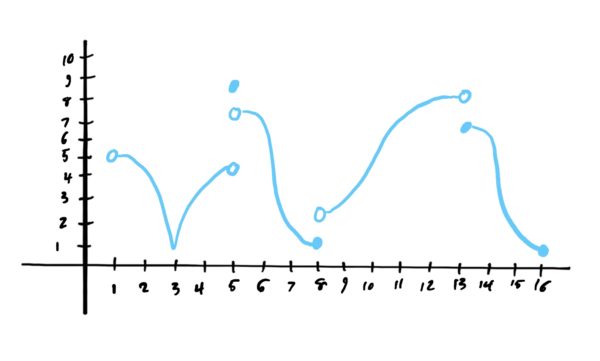
7. (Bonus +5) Find all local mins and maxes of \(h\).
8. (Bonus +5) What values does \(h\) attain at each of these local extrema?
9. (Bonus +5) What are the global maxes (if any)? What are the global mins (if any)?

Find all critical points for the following functions, and classify each critical point as a local min, max, or neither.

10. \(f(x)=x^3-6x^2+5x-2\)
11. \(g(x)=-2.1x^3-8.52x^2+5.4x\)
12. \(h(x)=x^5-2\)

Find the global min and max for the following functions on the given interval.

13. \(g(x)=x^2+2x+4\) on the interval \([-3,1]\)
14. \(f(x)=x^3-6x^2+5x-2\) on the interval \([-1,4.5]\)

15. (Bonus +10) Find a function whose \(y\)-value at its global max(es) is the same as the \(y\)-value at its global min(s).